Math
Through mathematics at JCHS, students explore the connections that mathematics have to other academic disciplines and to problems from the real world.
Our approach to math instruction is informed by research by Dr. Jo Boaler and Dr. Peter Liljedahl, which has shown consistently that students learn the most when they are grappling with hard problems and feel some “productive discomfort.” While all math classes at JCHS include some traditional, direct instruction, our teachers emphasize students playing an active role in their own learning and engaging with unfamiliar, difficult problems.
Our math classes require students to lean into this productive discomfort, where students learn to think like mathematicians and explore unfamiliar concepts through discovery-based problem-solving. This requires students to embrace mistakes as essential to the learning process and work with peers along their journey.
- Interpret data using mathematical modeling in order to predict real world phenomena
- Work with graphical, algebraic, and numerical representations of a mathematical concept and understand the connections between those representations
- Communicate mathematics both orally and in well-written sentences
- Assess the reasonableness of numeric solutions to problems using estimation techniques
- Integrate procedural proficiency and conceptual understanding of mathematics
- Develop mathematical thinking and reasoning skills such as the ability to make conjectures and sound arguments
- Discover the relevance and importance of mathematics in their life
- Understand the limitations of mathematics
At-Level Math Classes: These classes focus on building foundational skills in the core high school mathematical disciplines of Elementary Algebra, Geometry, Advanced Algebra, Precalculus, and/or Statistics. These classes emphasize procedural fluency and repeated practice of those skills that will most critically serve students in college and beyond. These classes also move at a more measured pace and ensure students are prepared for the next level class.
These classes emphasize the development of a “mathematical mindset” by introducing students to unfamiliar problems on a regular basis. Students work with peers on discovery-based problem-solving activities with direct teacher support.
AP and Advanced Math Classes: These classes aim to help students build advanced problem-solving skills that they will need during and after AP Calculus, in particular those students who aspire to highly selective STEM programs. While these classes do cover more material and move at a more rapid pace than the corresponding at-level class, the key difference is more one of attitude and approach.
These classes emphasize the development of a “mathematical mindset” by challenging students to tackle difficult and unfamiliar problems on a daily basis. In small groups, students learn to explore new topics with minimal direction from the teacher. Initially, this often feels challenging because it requires students to think deeply and be open to trying different approaches than those that they may have used in previous classes.
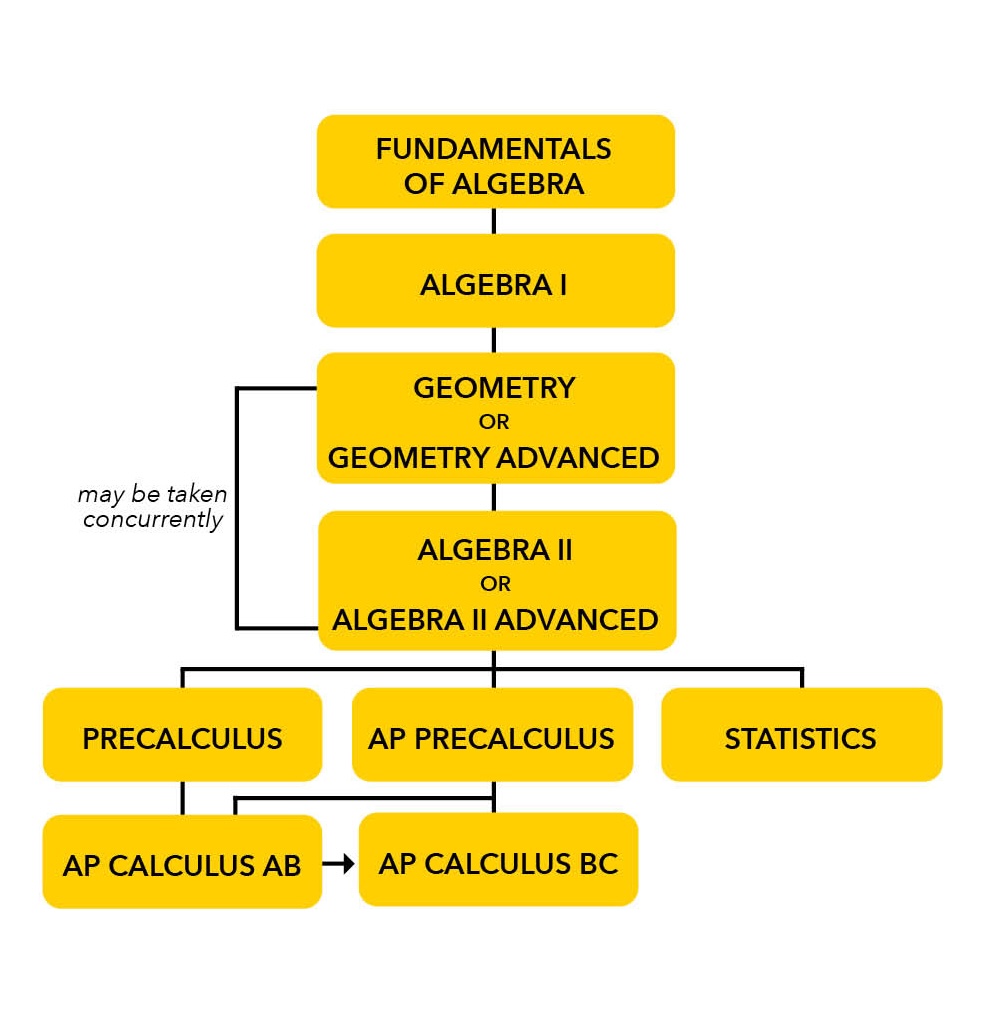
Math Requirements: All students must complete three years of core math courses through at least Algebra II. A course matching assessment is used to determine the appropriate level of math for each incoming student. Mastery of algebra is foundational for success in high school math and science, and many ninth graders at JCHS enroll in Algebra I. Students who excel in Algebra I and wish to reach AP Calculus in high school may take a summer Geometry class followed by Algebra II Advanced, or enroll in Geometry and Algebra II Advanced concurrently as 10th graders.
CORE COURSES
This small, highly personalized class is designed for students who need extra support mastering pre-algebra and middle school math concepts to provide a solid foundation for future success in Algebra I and other high school math classes. Students will work with hands-on mathematical tools such as algebra tiles to help conceptualize skills involving solving equations and all topics surrounding quadratic functions.
This highly differentiated course provides an in-depth exploration of algebra and allows all students to work at the edge of their math knowledge. Students will focus on interpreting how different relationships can be modeled while developing lateral thinking and in-depth problem-solving skills. Topics will include rates and ratios, algebraic expressions, linear equations, linear functions, systems of equations, quadratics, and exponents and radicals.
Prerequisite(s): Algebra I
This course is an introduction to Euclidean geometry. There is an emphasis on developing a student’s understanding of mathematical theory, problem solving, and the ability to write clear, logical arguments based on definitions, postulates, and theorems. Geometry is explored through many modalities, including algebra, formal and informal proof, compass construction, architecture, and origami.
Prerequisite(s): Algebra I and Department Approval
This course offers an in-depth study of the properties and applications of common geometric figures in both two and three dimensions, where students will engage in the study of transformations and right triangle trigonometry, employing both inductive and deductive thinking skills in problem-solving situations. Additionally, the course places a strong emphasis on writing proofs to solve and prove properties of geometric figures, fostering a deep understanding of Euclidean Geometry. Complementing this traditional focus, we also introduce elements of non-Euclidean geometries, dedicating a portion of our curriculum to exploring innovative concepts like Finite Affine Geometry and Projective Geometry through interactive games like SET and Spot It!. This blend of traditional and modern geometrical studies not only solidifies a strong foundation in mathematical concepts but also encourages creative thinking and a deeper appreciation for the subject’s complexity and beauty, preparing students for advanced mathematical pathways.
Prerequisite(s): Geometry
This course focuses on developing systematic understanding of functions through graphing, developing number sense, representing and solving functions, deepening algebraic manipulation skills, and solving related word problems. Topics include linear functions, quadratics, transformations, complex numbers, polynomials, rational equations, and exponential and logarithmic functions. Students grow in their capacity to puzzle through unfamiliar problems, with an emphasis on real-world applications of the mathematical concepts they are studying.
Prerequisite(s): Geometry Advanced or (Geometry and Department Approval) or (Algebra I with concurrent enrollment in Geometry and Department Approval)
This course covers the same content as Algebra II, but more theory and open-ended problem solving will be emphasized. Students will be challenged to use their reasoning skills, deepen their mathematical practices, and work together to solve hard problems. The class encourages creative thinking and a deeper appreciation for the subject’s complexity and beauty, preparing students for AP Precalculus and AP Calculus.
Prerequisite(s): Algebra II (C or higher)
This course is an in-depth treatment of the mathematics essential to Calculus with an emphasis on trigonometry. Topics include the Cartesian plane; polynomial, rational, exponential, logarithmic, inverse, circular, and trigonometric functions; and solving inequalities and systems of equations. Additional topics may include matrices, determinants and vectors. A strong focus on real-world problems solving in relation to the various mathematical topics covered will also be present.
Prerequisite(s): Algebra II Advanced or (Algebra II and Department Approval)
Students will explore more deeply many of the algebra concepts they have already seen such as: function notation, domain/range, rational expressions, logs and exponentials, and trigonometry while also learning new topics like matrices, sequences/series, conic sections, polar/parametric equations, and limits. A very strong emphasis will be placed on using the tools of Algebra I & II and Geometry to untangle complex problems so that students are well prepared for the AP Calculus curriculum. Students will be working on both developing a vision for untangling these problems and improving their consistency in applying the rules of algebra. Second semester students will apply their content understanding of conic sections, parametric equations, trigonometry, and matrices to do a project on modeling orbits in our solar system on Desmos, an online graphing tool. This course will also directly practice the math used in AP Chemistry, AP Biology, and AP Physics
Please Note: AP courses may require additional meeting times throughout the year.
Prerequisite(s): AP Precalculus or (Precalculus and Department Approval)
This course is an introduction to differentiation and integration of functions of a single variable. Topics include functions, limits, continuity, differentiation, related rates, min-max problems, graphing, integration and applications. Students will both work independently and in small groups to try out new problem forms and cover all the topics that will prepare them for the AP exam.
Please Note: AP courses may require additional meeting times throughout the year.
Prerequisite(s): AP Calculus AB or (AP Precalculus and Department Approval)
This one-year BC Calculus course offers an in-depth exploration of advanced calculus topics. It covers differential and integral calculus of single-variable functions, including the study of limits, derivatives, and integrals. Students will delve into parametric, polar, and vector functions, and examine polynomial approximations and series. The curriculum extends to advanced integration techniques, sequences, and series, including Taylor and Maclaurin series. Emphasizing a thorough understanding of calculus concepts, the course prepares students for rigorous mathematical applications, making it ideal for those pursuing mathematics or science-related fields.
Please Note: AP courses may require additional meeting times throughout the year.
Prerequisite(s): Algebra II
Statistics is an extremely powerful and often misunderstood mathematical tool which offers many applications to analyzing the real world. This course emphasizes a real-world data, project-based approach. Students will learn how to collect, research, summarize, display, analyze, and communicate written conclusions about data. Students will have opportunities to both do statistical analyses on data and think critically about statistical interpretations and detect flaws in statistical reasoning. Students will also learn to use spreadsheets as a computational tool. The class will focus primarily on statistics and probability, but will also include a final component of real world numerical applications, including learning to do basic taxes, selecting better credit cards, developing a budget, and learning about the time value of money. These other mathematical applications will be covered in a combination of in class learning and individual projects to learn about a selected area of interest.
Please Note: Students who plan to take both Precalculus and Statistics are strongly encouraged to take Precalculus first, immediately upon completion of Algebra II.
MATH COURSE SEQUENCE